Analyzing nonlinear structural behavior is a crucial aspect of civil engineering design, particularly when you’re confronted with intricate materials, significant deformations, or dynamic loads. Nonlinear behavior crops up when a structure’s response isn’t directly proportional to the applied load. This can happen due to factors such as a changing stiffness or strength in response to load history. Understanding and addressing these nonlinear aspects is pivotal in optimizing the performance, safety, and reliability of your designs. In this discussion, we’ll delve into the methods and concepts for examining nonlinear structural behavior in a manner that resonates with construction professionals.

Nonlinear Material Models
Nonlinear structural behavior often springs from the material used. Different materials exhibit varying stress-strain relationships, which can be linear or nonlinear, elastic or inelastic, isotropic or anisotropic, and so on. To account for these variations, it’s essential to employ appropriate material models that accurately represent the material’s specific characteristics. For instance, metals might require plasticity models, while rubber would demand hyperelastic models. Concrete, on the other hand, may necessitate damage models. Additionally, you have the flexibility to create your own material behavior models by using user-defined data or experimental insights.

Nonlinear Geometry
The geometry of a structure can also introduce nonlinearity, particularly when it undergoes significant deformations. This alters its size and shape, leading to changes in stiffness and load-carrying capacity. We categorize this as geometric nonlinearity, which can be divided into two types: material nonlinearity and follower nonlinearity. Material nonlinearity occurs when the structure’s deformation changes the distribution of stresses and strains within the material. This, in turn, leads to nonlinear material behavior. Follower nonlinearity emerges when the direction of the applied load shifts along with the structure’s deformation, resulting in additional moments and forces. To tackle geometric nonlinearity in your designs, you’ll need to employ nonlinear finite element methods that continually adjust the configuration and equilibrium of the structure as the load increases.

Nonlinear Boundary Conditions
The boundary conditions of a structure present a third avenue for nonlinearity. These conditions dictate how the structure is supported, restrained, or linked to other elements. Depending on the type and placement of these conditions, they can introduce nonlinear elements to the structural behavior. For instance, a hinge support can introduce nonlinearity by allowing rotation but not translation, a gap element can introduce nonlinearity by enabling contact or separation, and a spring element can introduce nonlinearity through its nonlinear stiffness. When addressing nonlinear boundary conditions in your designs, you must integrate nonlinear elements or constraints that faithfully replicate the behavior of these boundary conditions.

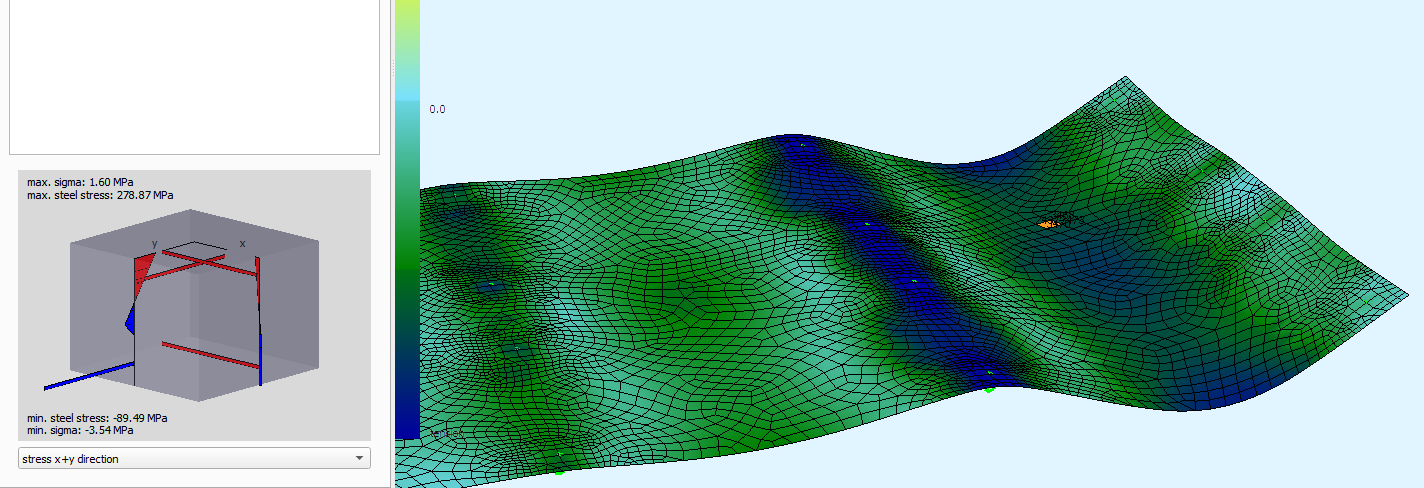
Nonlinear Loading

Another key factor contributing to nonlinearity in structural behavior is the way a structure is loaded. Loading can come in various forms – it can be static or dynamic, consistent or variable, predictable or random, and so on. Depending on the characteristics and intensity of the load, it can induce nonlinearity in how the structure behaves. For example, a static load can introduce nonlinearity when it pushes the material beyond its elastic limit. On the other hand, dynamic loading can create nonlinearity by introducing inertia and damping effects. Variable loads can cause nonlinearity by leaving a historical impact, and random loads can result in nonlinearity due to their stochastic nature. To tackle these nonlinear loading scenarios in your designs, you must apply nonlinear analysis techniques that break down the loading into increments or steps and continuously monitor the solution’s convergence and stability.

Nonlinear Solution Methods

To unravel and understand nonlinear structural behavior in your designs, you need to employ nonlinear solution methods capable of managing the intricacies and diversity of nonlinear influences. These methods are numerical techniques that systematically solve the nonlinear equations of equilibrium and structural compatibility. Various types of nonlinear solution methods are at your disposal, including the Newton-Raphson method, modified Newton-Raphson method, arc-length method, and load control methods. Each method comes with its own set of advantages and disadvantages, so it’s crucial to select the one that best aligns with your specific problem. Additionally, you’ll need to consider certain factors and criteria that impact solution accuracy and convergence, like the size of the load increments, tolerance levels, iteration limits, and error assessment norms.

Nonlinear Output and Interpretation

Once you’ve completed the nonlinear analysis of your designs, the next step is interpreting the output and results of the analysis. The output of a nonlinear analysis encompasses data like displacements, stresses, strains, reactions, forces, moments, and energy of the structure. To make sense of this data, you can utilize a variety of tools and techniques, such as graphs, tables, charts, contours, animations, or relevant indicators. It’s also valuable to compare your findings with the expected or desired outcomes, which may include design criteria, adherence to industry codes and standards, or comparisons against experimental data. Furthermore, you can delve into sensitivity and parametric studies to explore the impact of different variables or parameters on the nonlinear structural behavior of your designs. This helps in fine-tuning your construction plans to meet the desired standards and specifications.

Conclusion

In the complex world of civil engineering design, understanding and managing nonlinear structural behavior is pivotal. This multifaceted phenomenon arises from a variety of sources, including material properties, geometric changes, boundary conditions, and loading characteristics. To tackle these challenges, professionals need to employ advanced nonlinear analysis methods, carefully selecting appropriate material models, and navigating complex solution methods. Furthermore, interpreting the results and conducting thorough evaluations are essential steps in ensuring the reliability and safety of our designs. By delving into the intricacies of nonlinear behavior, we empower ourselves to construct structures that stand the test of time, meeting the highest standards of performance and safety.